¶ Theoretical Considerations in Absorbance Measurements
¶ Ideal Case: Beer-Lambert Law
The Beer-Lambert Law is a fundamental principle in spectroscopy that relates the absorbance of light by a sample to its concentration and the path length through which the light travels
- The power of the Beer-Lambert Law originates from its linearity
- By measuring the absorbance of a sample at a specific wavelength, one can determine the concentration of the absorbing species within the sample, provided the molar absorption coefficient is known
The Beer-Lambert Law makes several assumptions to ensure its validity. These assumptions include:
- The incident light is perfectly monochromatic, meaning it consists of a single wavelength
- The absorbing species in the sample act independently of each other, without any significant interactions or interferences
- The incident light beam is perpendicular to the surface of the absorbing medium, minimizing any angle-dependent effects
- The path length traversed by the light beam is uniform across the entire sample, allowing for accurate measurements
- The absorbing medium does not scatter the incident light, ensuring that all the detected light is solely due to absorption.
¶ Deviations from the Ideal Case
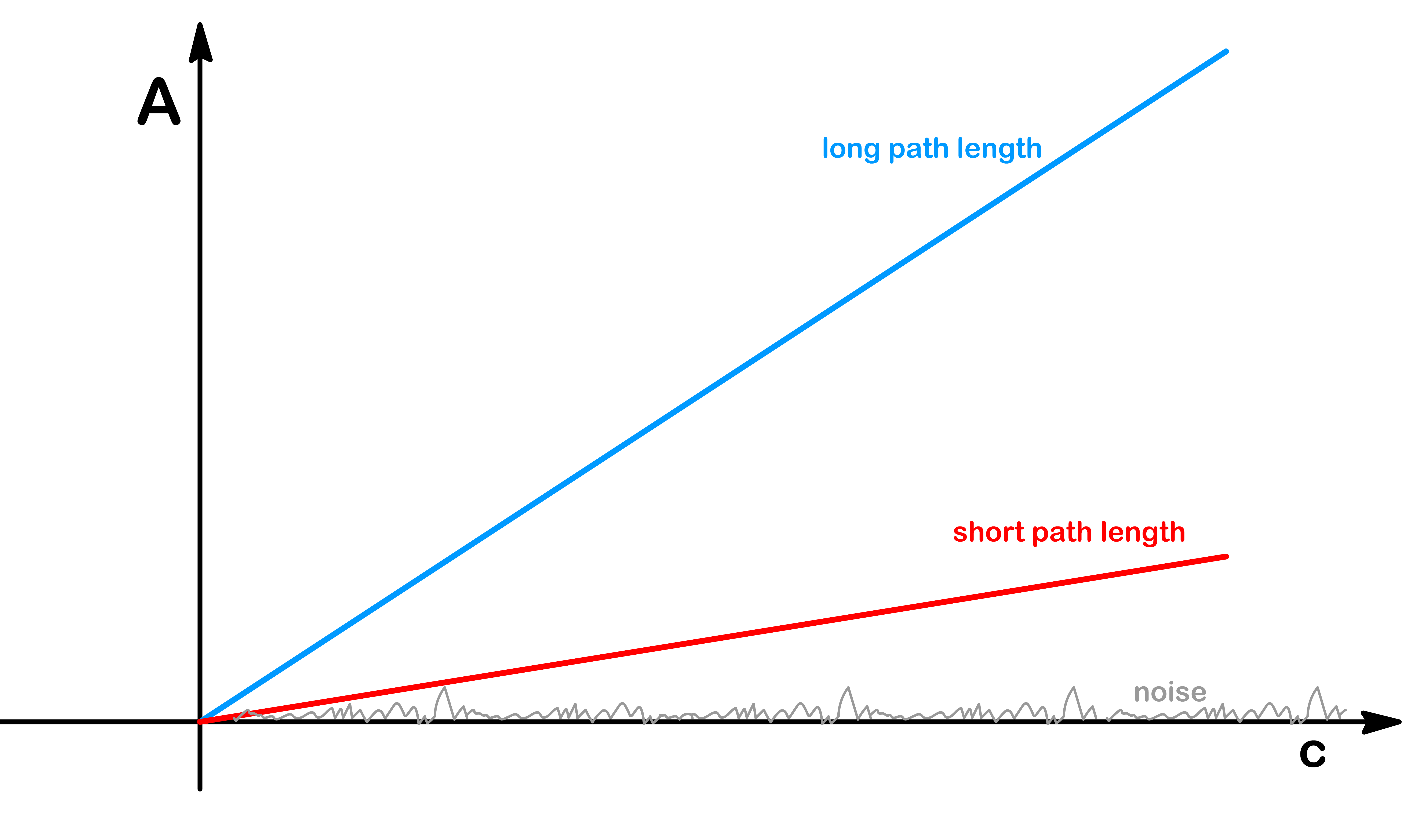
Path Length Dependence
In many cases, the path length can be assumed to be linear as long as the container holding the sample has a well-defined shape and size
- This linearity assumption simplifies the calculations and facilitates the interpretation of the absorbance measurements
By using a longer path length, the absorbance value (A) becomes larger
- This is advantageous because a larger absorbance value provides a greater dynamic range, allowing the detected signal to be further away from any potential noise present in the system
- As a result, the signal-to-noise ratio improves, enhancing the accuracy and precision of the absorbance measurements
In cases of high concentration where the non-linearity of the Beer-Lambert Law arises from excessively high absorbance, adjustments to the path length may be necessary to maintain linearity
- In such situations, decreasing the path length can effectively bring the absorbance back into the linear regime
- This approach allows for accurate determinations even at high concentrations where non-linear effects become prominent
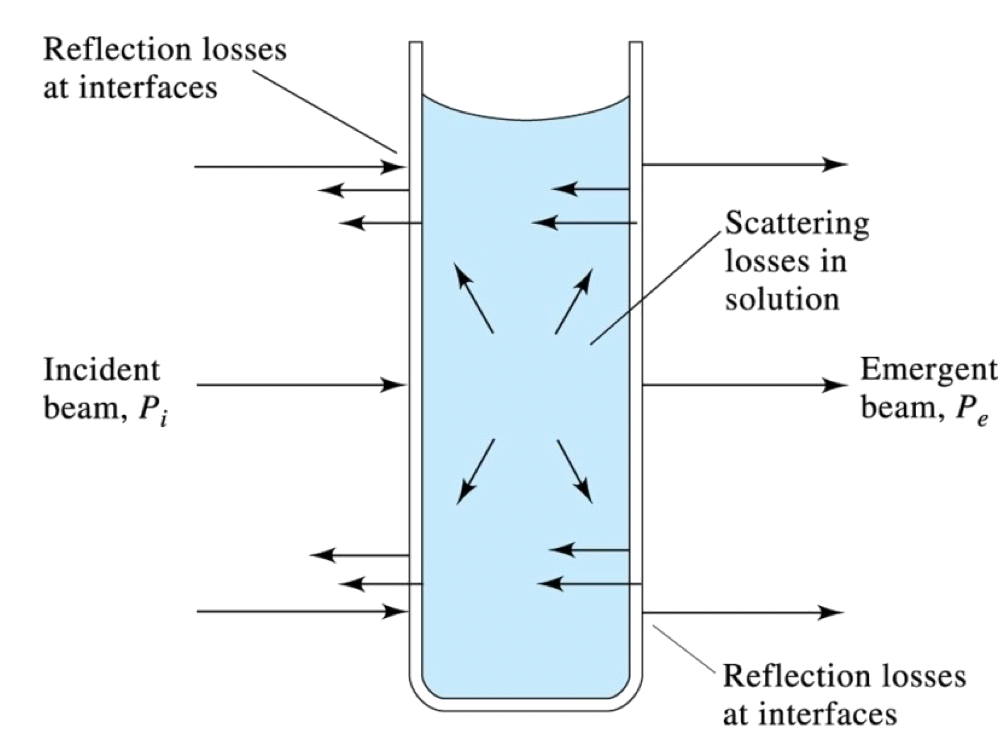
Scattering of Light
It is important to note that in addition to absorption, light can also be scattered by particles or molecules present in the sample
- When light passes through a sample, it can interact with particles or molecules in the sample, leading to scattering
- Scattering occurs when the incident light is deflected in different directions by these scattering centers
This scattering phenomenon can influence the measured intensity and affect the accuracy of the absorbance measurements
- is the intensity of the scattered light
- This scattered light can be detected along with the transmitted light, contributing to the measured intensity, so it is also included in the numerator
Molar Absorption Coefficient and Refractive Index
The refractive index () is a fundamental optical property of a material that describes how light propagates through it
- It is defined as the ratio of the speed of light in a vacuum to the speed of light in the medium. In other words, it quantifies how much the velocity of light is reduced when it passes through the material
- The refractive index is influenced by various factors, including the density and composition of the material. Hence, changes with the concentration of the solution
It turns out the molar absorptivity depends on the index of refraction
- This shows the relationship between the molar absorption coefficient corrected for refractive index () and the individual molar absorption coefficient () and refractive index ()
- Since refractive index is dependent on concentration, the corrected molar absorption coefficient will also be dependent on concentration of the solution
- The deviation is often not very large and rarely significant at concentrations below 0.01 M
Concentration-Dependent Factors
When the concentration of a substance increases, the interactions between molecules become more pronounced
- The presence of strong intermolecular forces, such as hydrogen bonding or van der Waals interactions, can cause changes in the absorption properties of the molecules
- These interactions can alter the electronic states or energy levels of the molecules, resulting in nonlinearity in the absorption response.
Changes in concentration can also lead to shifts in chemical equilibrium
- In some cases, an increase in concentration can affect the equilibrium between different chemical species or the distribution of molecules between different states
- These shifts in equilibrium can cause variations in the absorption behavior, leading to nonlinearity in the measured absorption spectra
¶ Uncertainty in the Measurement
The relative concentration uncertainty can be described by the following equation
- The standard deviation of the concentration, denoted as , is directly related to the transmission and the standard deviation of the transmission,
- The relationship between these variables depends on different cases of uncertainty sources
Case A
- The uncertainty is independent of T and is primarily caused by limited readout resolution
- In this case, the standard deviation of the transmission is constant, represented as
Case B
- The uncertainty is dependent on and arises from photon detector shot noise
- The standard deviation of the transmission is given by
Case C
- The uncertainty is directly proportional to T and is influenced by factors such as cell positioning and flicker noise
- The standard deviation of the transmission is expressed as
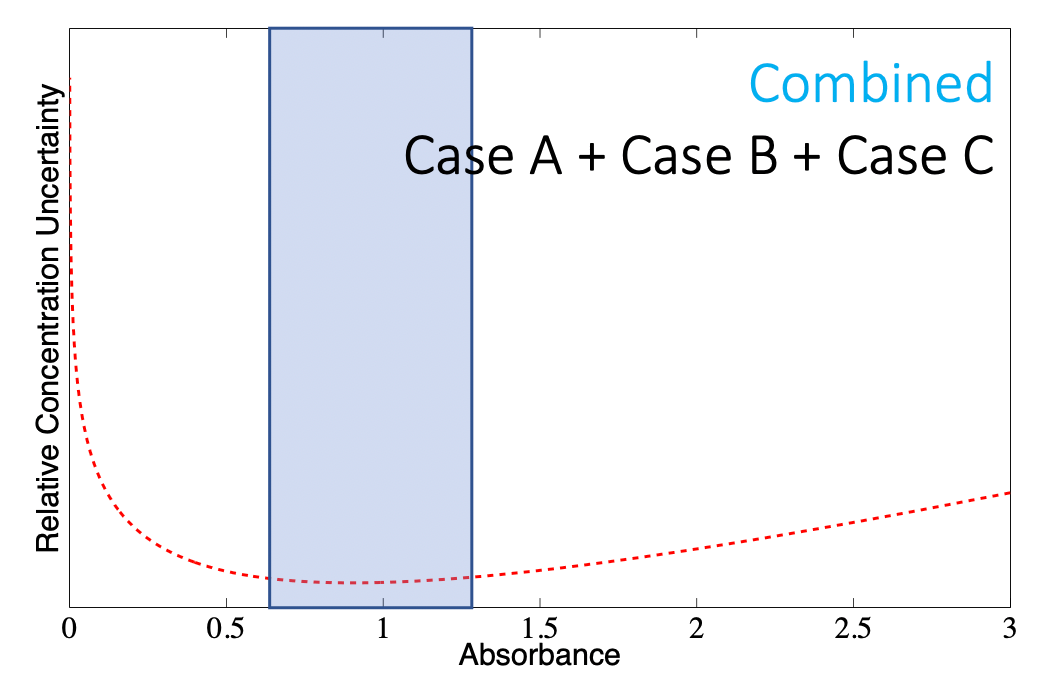
In practice, the overall concentration uncertainty is a combination of the uncertainties from all three cases
- It is the sum of Case A, Case B, and Case C uncertainties
- Each case contributes differently depending on the specific experimental setup and the dominant sources of uncertainty
- is usually minimized when is near 1, which is why we usually calibrate to 1
¶ Instrumentation
¶ Light Source
In absorbance measurements, it is often necessary to use two lamps
- A UV source for ultraviolet measurements and a visible source for measurements in the visible range
- Both light sources must meet certain requirements to ensure accurate and reliable measurements.
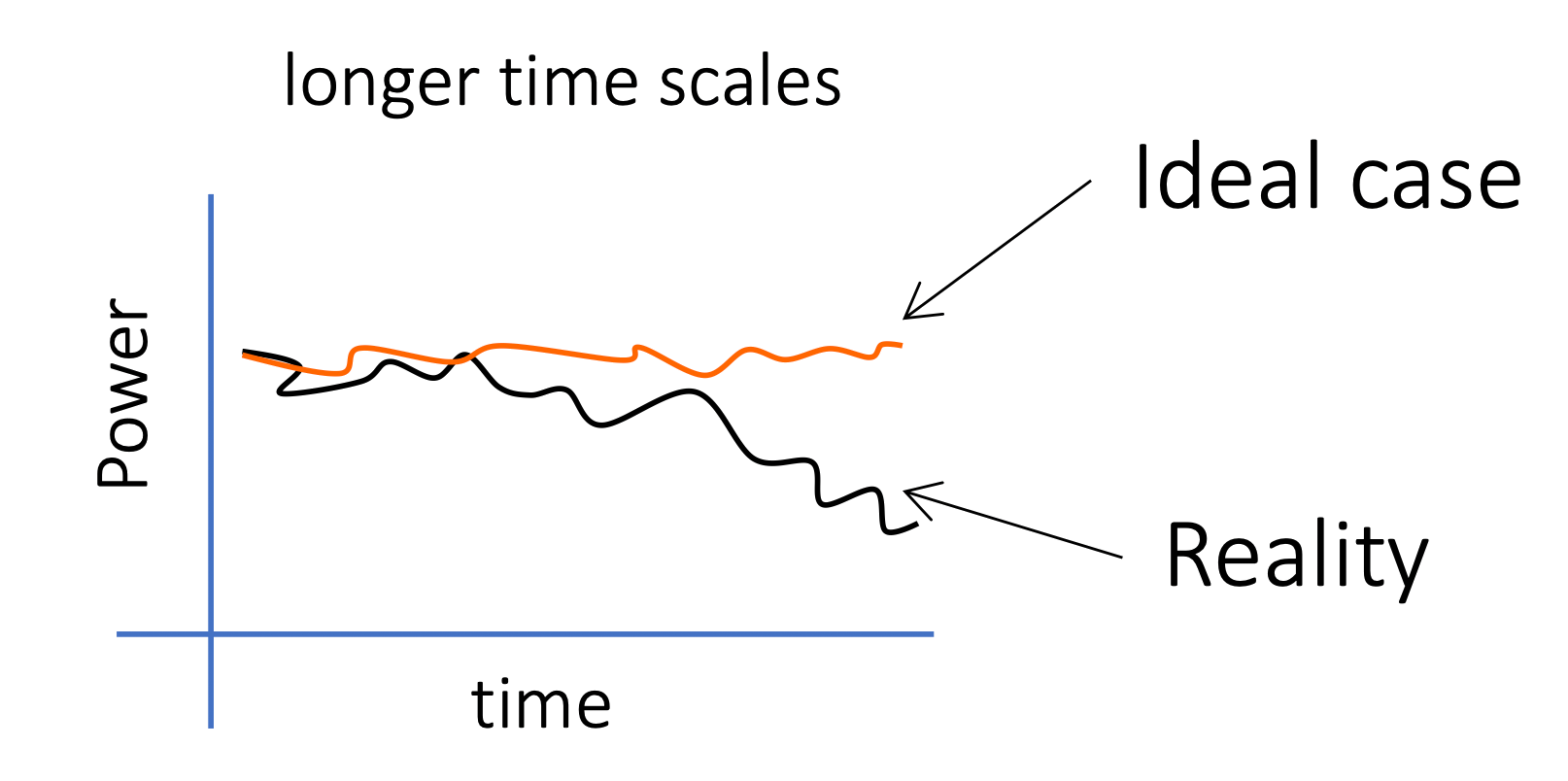
Stability
Stability refers to the ability of the light sources to maintain a constant output intensity over time
- Fluctuations or instabilities in the light source can introduce variability in the measured absorbance values, leading to inaccuracies in the results
Light sources are usually stable over shorter time scales, but unstable over longer time scales
- We can run multiple samples over short time scales without recalibrating
- We need to constantly calibrate it to correct for fluctuation in Power if we are running the same over a long time scale
Approximation of Black Body Source
A black body source is an ideal theoretical emitter that emits radiation at all wavelengths
- While it is not possible to achieve a perfect black body source, the light sources used in absorbance measurements should approximate this behavior as closely as possible
By approximating a black body source, the light sources emit a broad range of wavelengths, allowing for comprehensive spectral coverage
- This is essential because absorbance measurements may involve samples that absorb light at different wavelengths
- A light source that approximates a black body source provides a continuous spectrum of light, ensuring that all the relevant wavelengths are covered, and enabling accurate measurements across the entire spectral range of interest
¶ Wavelength Selector
In spectroscopic measurements, a wavelength selector is employed to isolate a specific wavelength or a narrow range of wavelengths for analysis
- There are two common types of wavelength selectors: optical filters and monochromators, each with its own characteristics and applications
Optical filters
Optical filters are widely used as wavelength selectors in various spectroscopic techniques
- They are designed to transmit light within a specific wavelength range while blocking or attenuating light outside that range
To fulfill the assumption of the Beer-Lambert Law, optical filters used as wavelength selectors should possess high monochromaticity
- Monochromaticity refers to the ability of the filter to transmit light within a narrow wavelength band, minimizing any spectral leakage or contamination from neighboring wavelengths
- By selecting an appropriate optical filter, we can isolate the desired wavelength range for absorbance measurements
Optical filters come in various forms and sizes, offering flexibility in terms of wavelength range and application
- They are relatively simple to use and provide a cost-effective solution for wavelength selection in many spectroscopic setups.
Monochromators
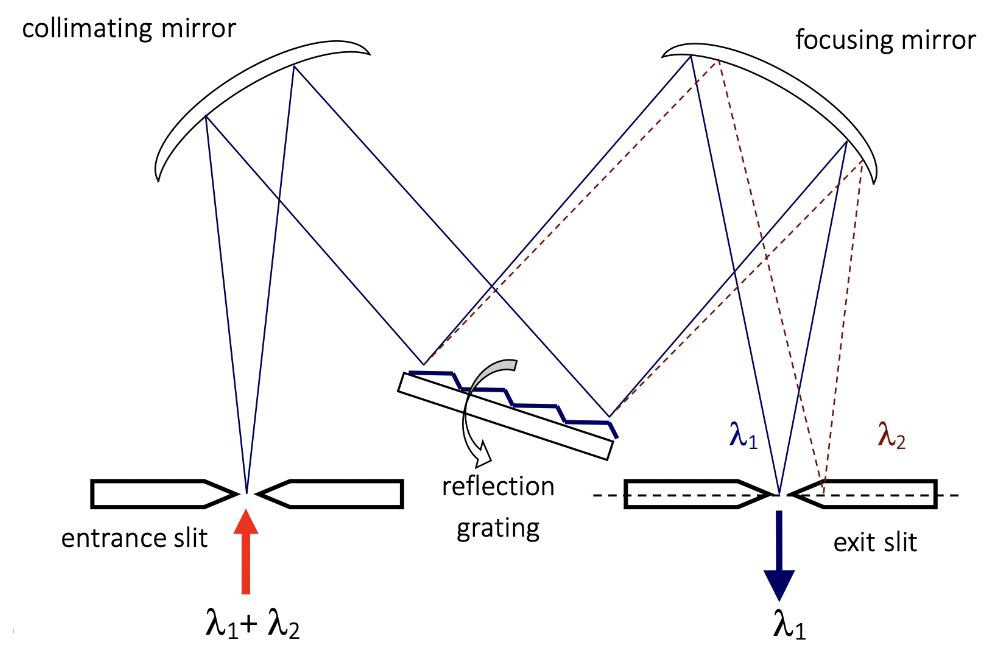
Monochromators utilize the principle of dispersion to separate different wavelengths spatially
- The basic structure of a monochromator includes an entrance slit, a dispersive element (often a grating), and an exit slit
Entrance slit
The entrance slit acts as a spatial filter, allowing only a portion of the incident light beam to pass through
- It is therefore responsible for controlling the intensity of light entering the monochromator
- The width of the entrance slit determines the effective bandwidth of the light entering the monochromator
- While the entrance slit is important for overall instrument performance, its precise adjustment is typically less critical compared to the exit slit
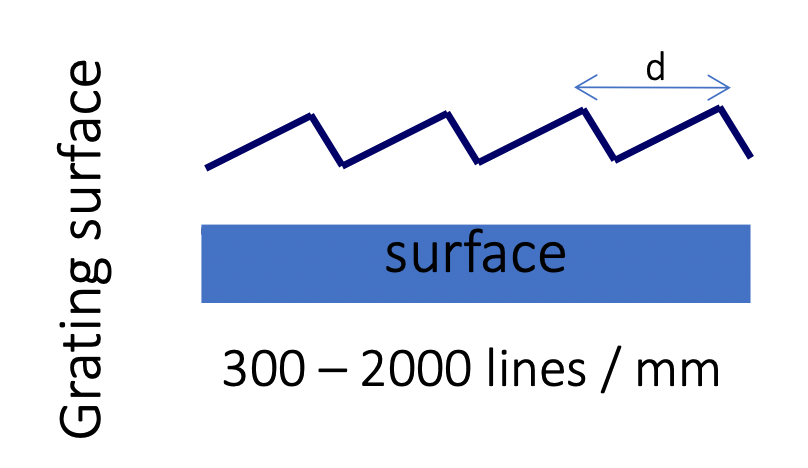
Dispersive Element
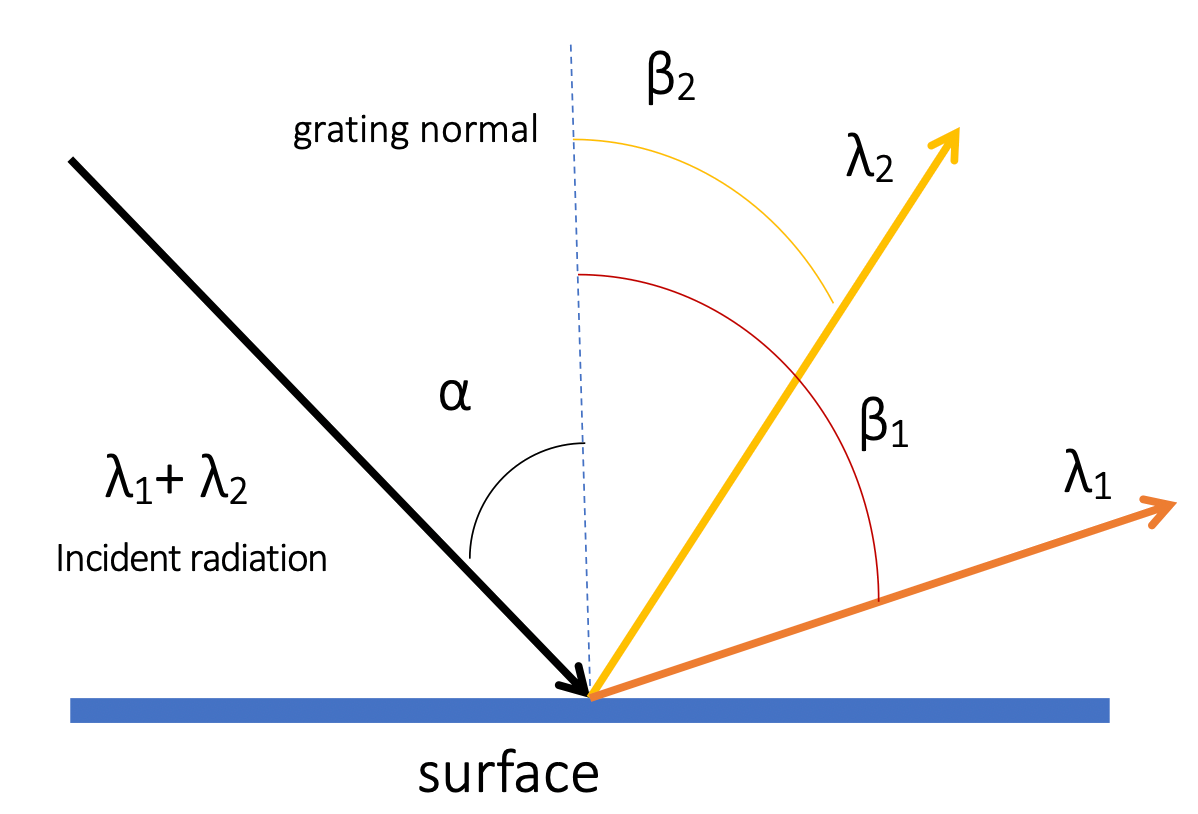
The dispersion phenomenon in monochromators occurs when light of different wavelengths impinges upon the grating
- As a result, the angle at which the light is diffracted changes depending on the wavelength
- This dispersion allows for the spatial separation of different wavelengths, enabling the selection of a specific wavelength or a narrow wavelength range
- Angle dependence on wavelength can be defined by a simple equation:
The angular dispersion ( ) of a monochromator determines the ability of the grating to separate different wavelengths effectively
- measures the range of angles into which a range of wavelengths is distributed
- The larger the angular dispersion, the better the grating performs in wavelength selection
- If is small ,then , so we can minimize to increase
Monochromators offer a high degree of flexibility in wavelength selection, allowing us to tune the output wavelength by adjusting the position of the exit slit
- They are particularly useful when precise control over the wavelength is required or when a wide range of wavelengths needs to be scanned
Exit Slit
The exit slit acts as a spectral filter, allowing only a narrow band of diffracted wavelengths to pass through to the detector
It determines the range of wavelengths that exit the instrument and strike the detector
- By adjusting the width of the exit slit, the desired wavelength range can be selected, effectively controlling the spectral resolution of the monochromator
The width of the slits plays a significant role in balancing the energy reaching the detector and the spectral resolution of the instrument
- A larger entrance slit width allows more light to enter the monochromator, increasing the overall signal level. However, it also permits a broader range of incident angles and wavelengths to reach the dispersive element
- A smaller entrance slit width reduces the amount of stray or scattered light reaching the dispersive element, which helps to improve the spectral purity and resolution. However, it also decreases the overall signal intensity reaching the detector, which can negatively impact the signal-to-noise ratio
¶ Considerations for Sample Holder Selection
The choice of a sample holder in spectroscopy is important to ensure accurate and reliable measurements
- Different materials, such as plastic, quartz, and glass, have their own advantages and limitations
Plastic Sample Holder
Pros :
- Cost-effective : Plastic sample holders are generally inexpensive, making them accessible for routine spectroscopic measurements
- UV transparency: Certain types of plastic materials can transmit ultraviolet (UV) light, allowing measurements in the UV range
Cons:
- Susceptible to scratches: Plastic is more prone to scratching, which can affect the quality of the transmitted light and introduce measurement artifacts
- Variability in transmittance (%T): Plastic materials may exhibit variations in their transparency, leading to inconsistent transmittance values and potential inaccuracies in the measurements
Quartz Sample Holder
Pros :
- UV compatibility: Quartz sample holders are suitable for UV spectroscopy, with good transmission properties down to 190 nm. This makes them ideal for applications that require measurements in the UV range
Cons:
- Cost: Quartz sample holders tend to be more expensive compared to plastic or glass options. The higher cost is attributed to the material's superior UV transparency and durability
Glass Sample Holder
Pros :
- Cost-effective: Glass sample holders are relatively inexpensive compared to quartz
- Wide spectral range: Glass sample holders typically provide good transparency down to approximately 350 nm, making them suitable for visible and near-UV spectroscopy applications
Cons:
- Limited UV performance: Glass sample holders may not be suitable for UV spectroscopy below 350 nm due to their limited transparency in the UV range. Measurements in this range may introduce significant absorption or scattering effects, leading to inaccurate results
¶ Considerations for Detector Selection
The signal of the detector is given by the equation
- is the signal
- is the sensitivity
- is the radiant power
- is the dark current
High Sensitivity
A high-sensitivity detector is desirable as it allows for the detection of low-intensity signals and improves the signal-to-noise ratio of the measurements
- The detector should be capable of detecting even weak signals and provide a reliable response over a wide dynamic range
Rapid Response
A detector with a rapid response time is desirable for capturing fast changes in the measured signal
- The response time should be fast enough to accurately capture the dynamics of the system under investigation
- The detector's response time is typically specified by its rise time or settling time
Wavelength Independence
For general spectroscopic applications, it is advantageous to use a detector that is wavelength-independent or has a consistent response across the desired spectral range
- This ensures that the detector's sensitivity and response do not vary significantly with different wavelengths, enabling accurate and reliable measurements throughout the spectrum of interest