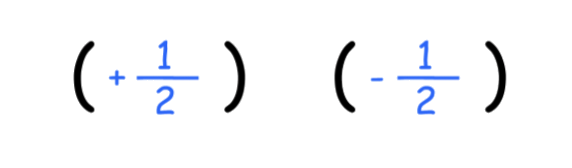¶ Nuclear Spin
A nuclear spin is related to the angular momentum of the nucleus, and is characterized by the symbol ( )
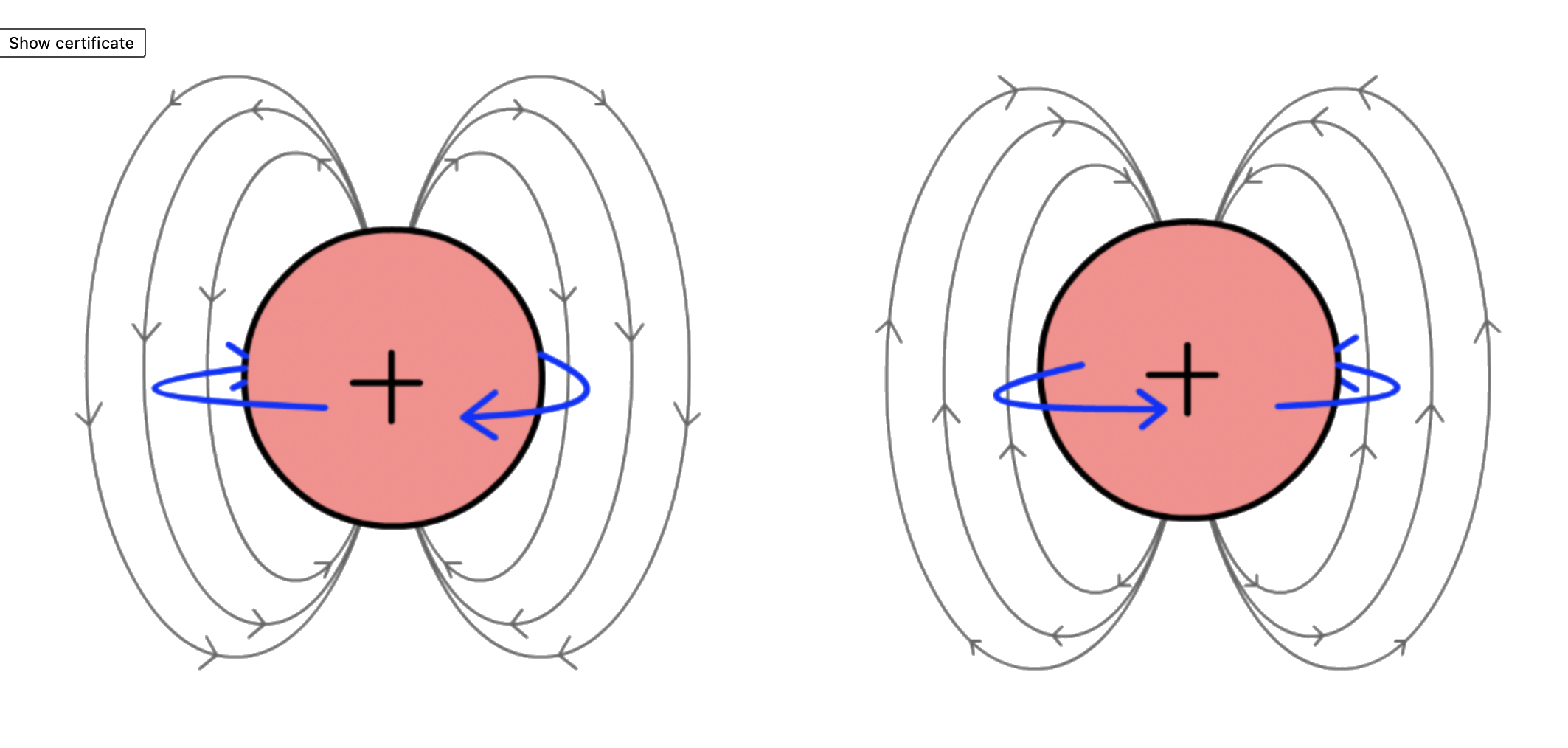
- When a charged particle spins, it will have an associated magnetic moment, which produces magnetic interaction with the environment
- We observe a similar phenomenon in particles like electrons and nucleus even though they are not actually physically spining, but since we are terrible at naming, we denote these intrinsic angular momentum as spins
- That said, with the loss of rigorousness, it does not really hurt to imagine the nuceleus as spining balls to illustrate certain properties
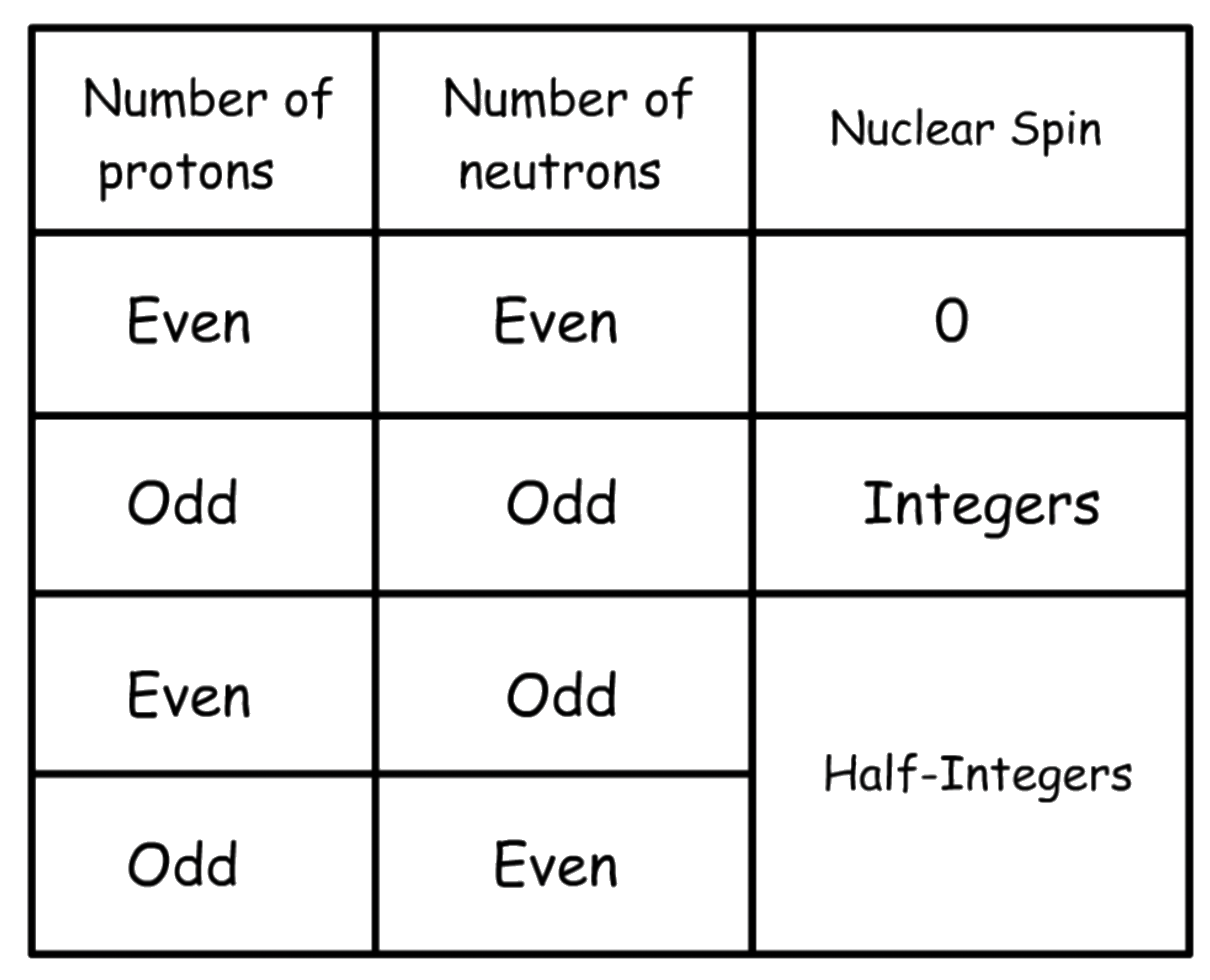
Nuclear spins are quantized, meaning only certain spin states and transitions are permitted
- The nuclear spin of a nucleus can be determined using this chart:
- Nuclei with I = 0 are NMR inactive, while nuclei with I > 0 are NMR active
The most important class of nuclei in NMR spectroscopy are those with spin because these nuclei have spherical distribution of charges
¶ Spin and Magnetic Field
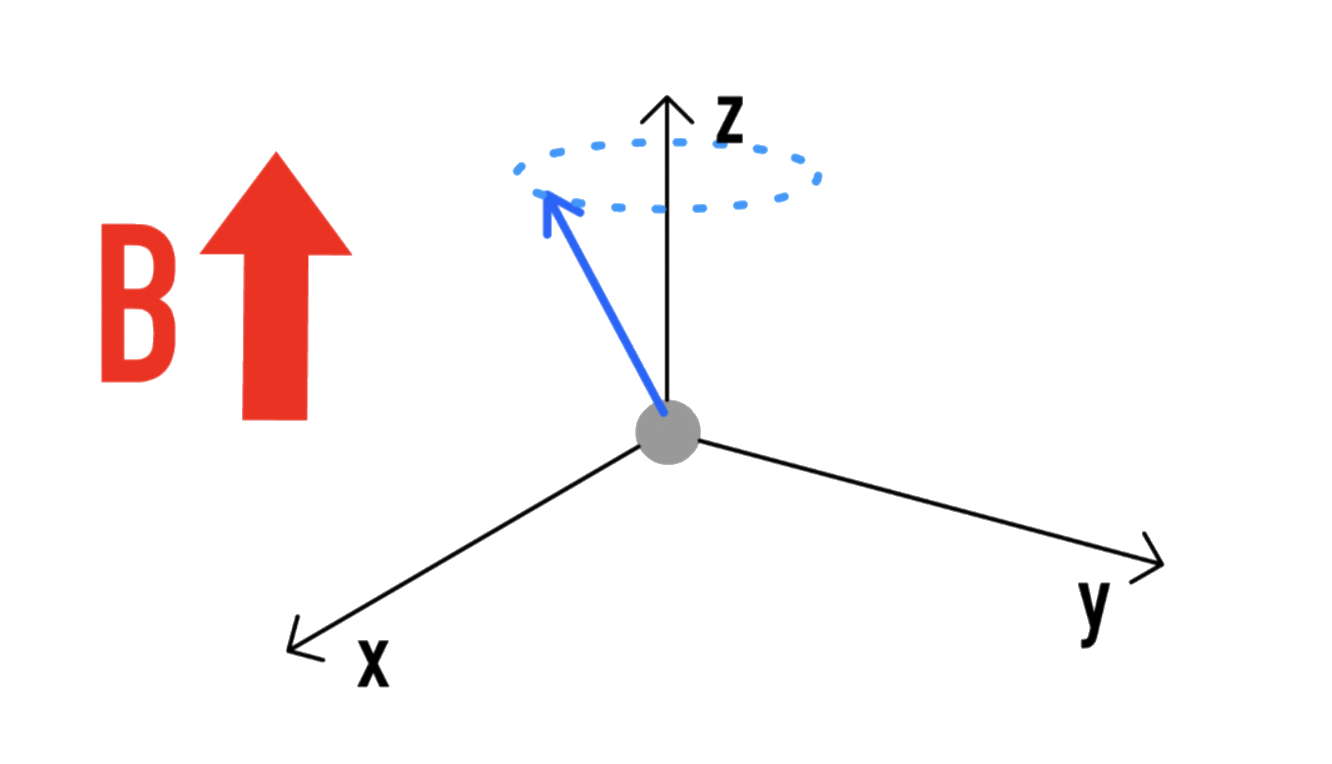
During NMR spectroscopy, a magnetic field will be applied onto the nucleus
- Much like how a spinning gyroscope will undergo precession in a gravitational field, a spinning charge will undergo precession under a magnetic field
- The frequency of precession is proportional to the strength of the magnetic field:
The proportionality constant is known as the gyromagnetic ratio and is proportional to the magnetic moment
- The gyromagnetic frequency ( ) is unique to the nucleus. Hence, each NMR experiment is tuned to its own individual nucleus
Spins are quantized, so the magnetic moment ( ) can only have certain quantized value
- There are values orientations of
- Hence, there are 2 orientations for nuclei : I=\pm\frac{1}
For all the nuclei that we are going to be concerned with, , although other values are possible
- we denote spin up ( ) as and spin down ( ) as
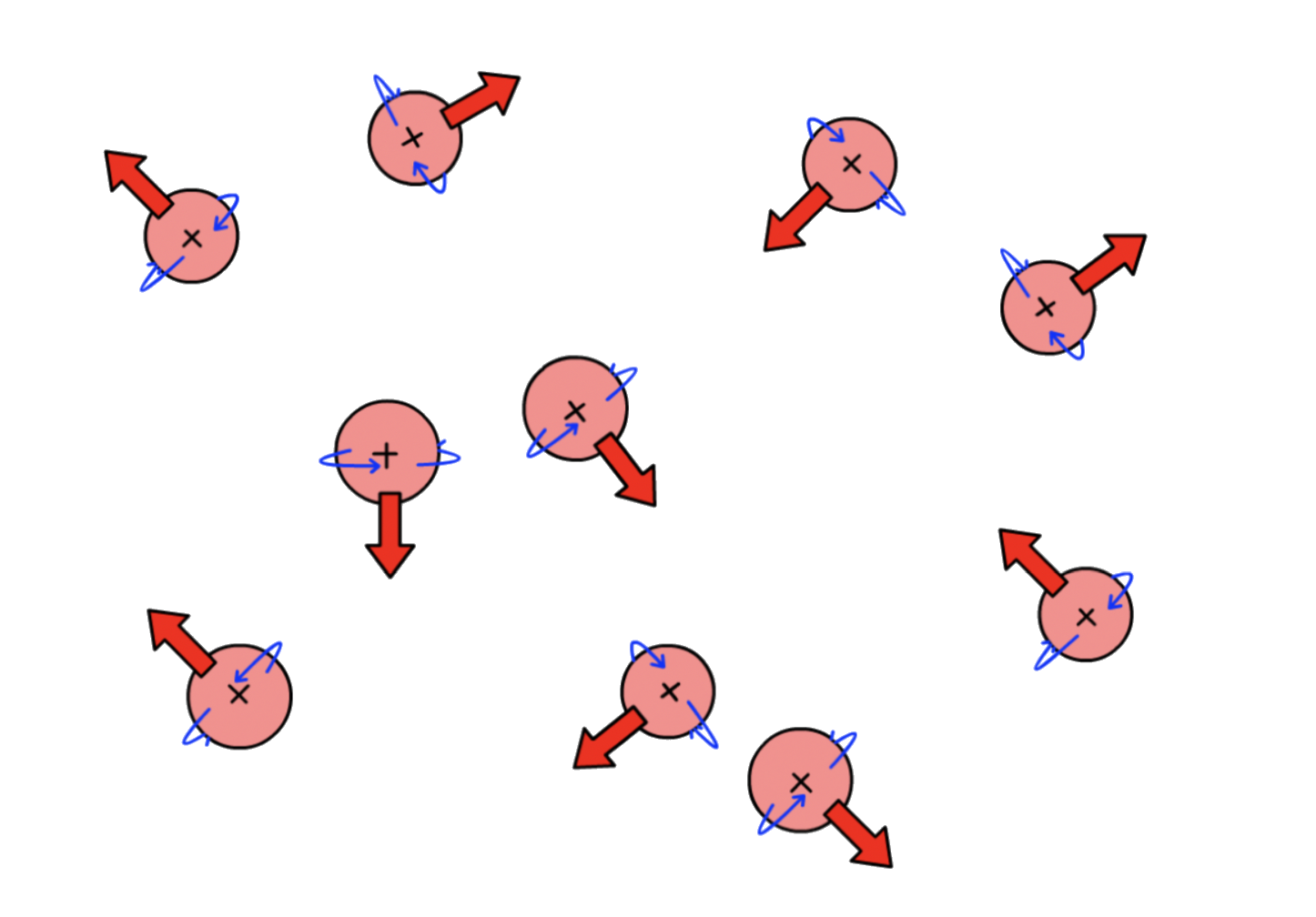
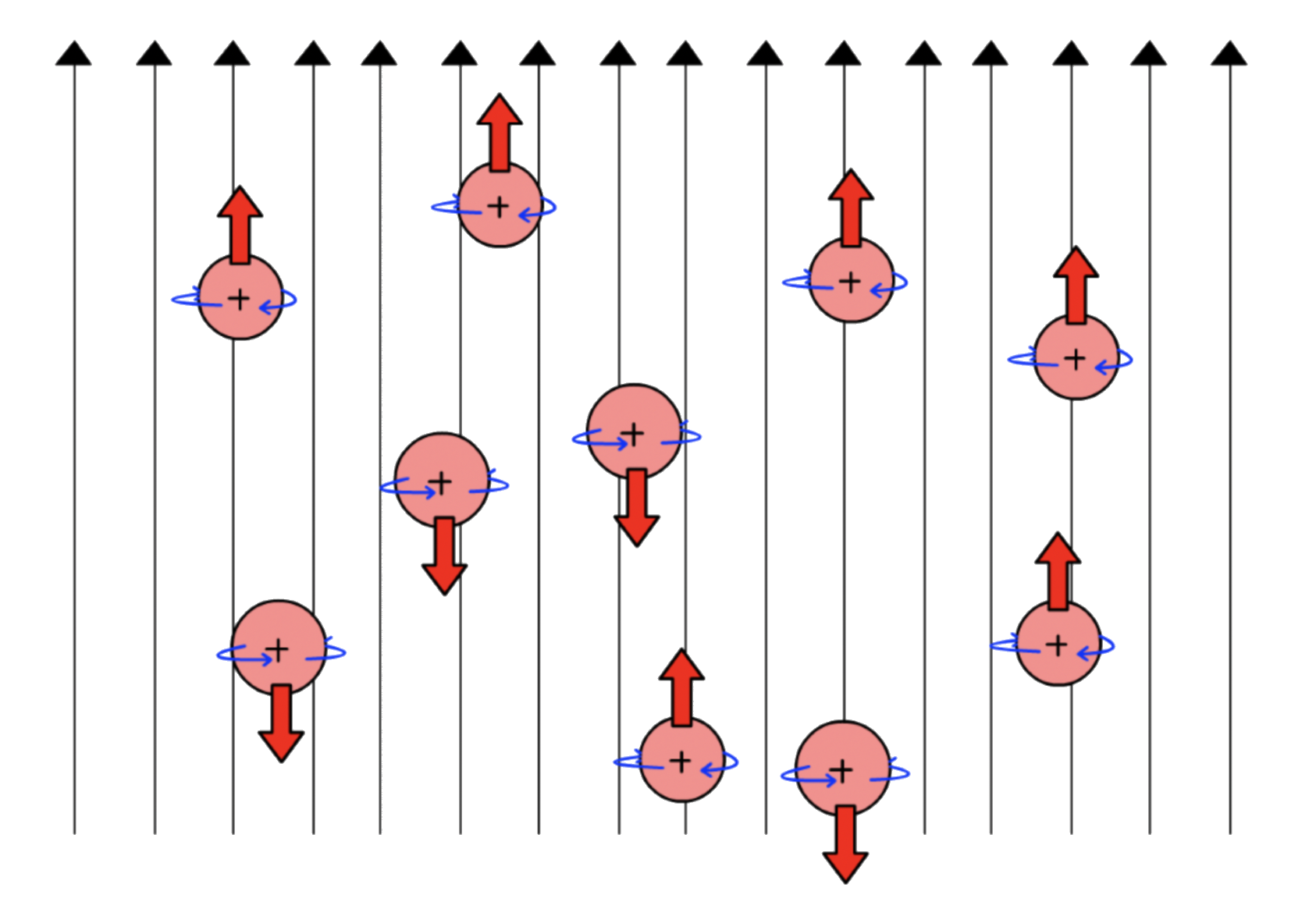
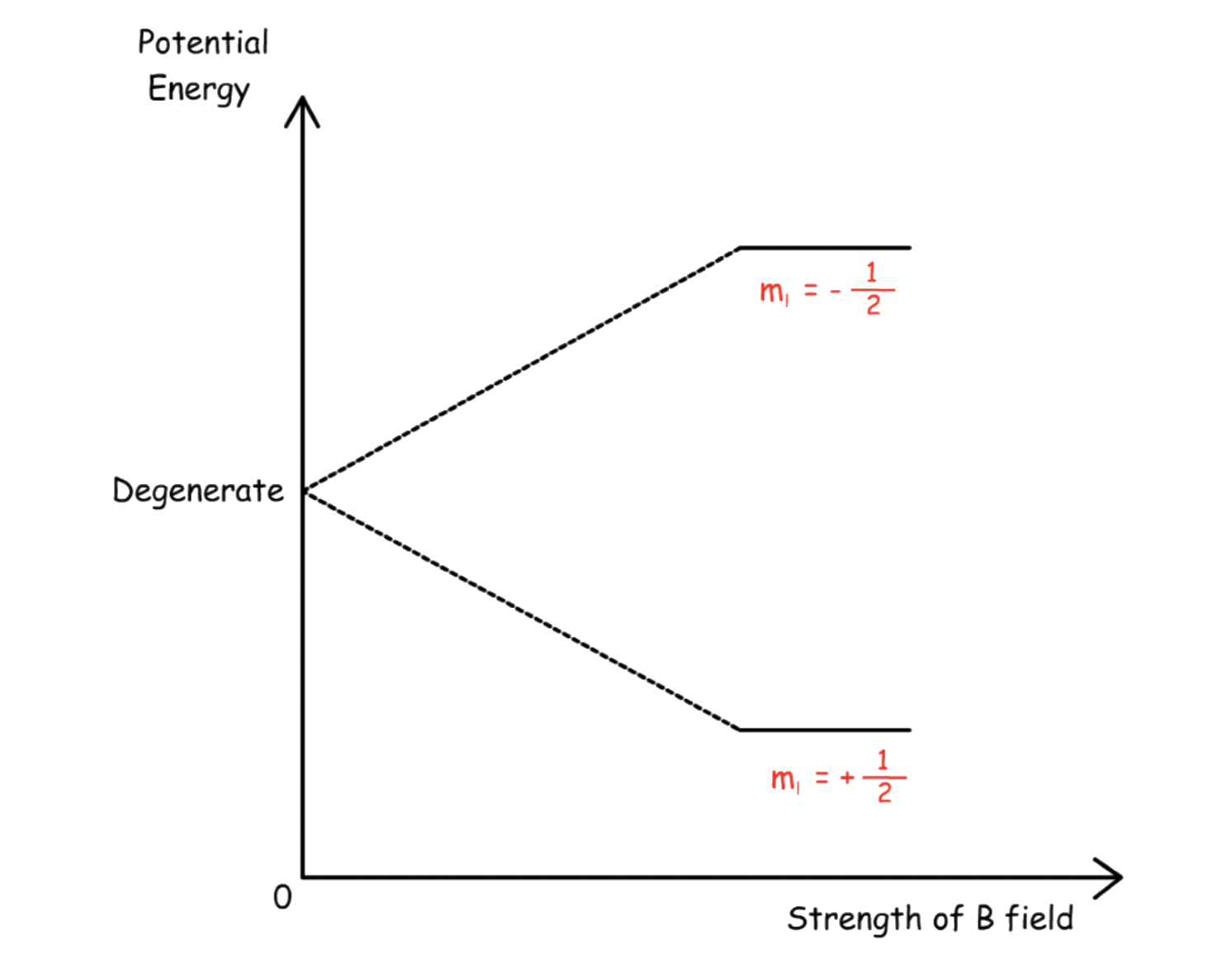
We can change the energy level of the system by applying a magnetic field
- In the absence of an applied magnetic field, the spins are randomly orientated and leading to degeneracy
- In an applied magnetic field, the spins are no longer degenerate and the nuclei will align along the field lines
The nuclei whose magnetic moment points in the same direction as the field lines are +½ ; They are stable and thus have a lower potential energy
The nuclei whose magnetic moment points in the opposite direction of the field lines are –½ ; They are unstable and thus have a higher potential energy
During NMR spectroscopy, radio wave are shone onto the sample in a magnetic field
- Radio wave with certain frequency will be absorbed to promote the nuclei from a low energy state ( along the field ) to a higher energy state ( against the field )
- The transition energy is directly proportional to the Larmor frequency
Selection rule
Only transitions in which m differs by one are allowed ( only one spin can be change at a time )
- A given photon (in the radio frequency range) can affect ("flip") only one of the two nuclear spins
¶ Chemical Shift
The resonance frequency of NMR absorption also depends on the chemical environment of that nucleus
- The origin of chemical shift can be traced to the electrons surrounding the nucleus, and the interactions of electrons with the applied magnetic field
¶ Factors resulting in chemical shifts
Two reasons that can result in chemical shifts:
- Ring currents caused by circulating electrons that generate a magnetic field
- Electronegativities of nearby atoms
Ring current
The delocalized electrons in a ring generates its own magnetic field.
- Outside the ring, this field is in the same direction as the externally applied magnetic field
- Inside the ring, the field counteracts the externally applied field
- As a result, the net magnetic field outside the ring is greater than the externally applied field alone, and is less inside the ring.
The direction of the ring current differs depending on the nature of the ring
- The direction of the ring current in an aromatic molecule is such, so as to generate a magnetic field that opposes the external field inside the ring (a ' diatropic ' current)
- The ring current in an antiaromatic molecule flows in the reverse direction (' paratropic ')
Hence, the nuclei within the ring will experience a diminished magnetic field, while the nuclei outside of the ring will feel an enhanced magnetic field
Electronegativity
The electrons orbiting the nucleus will generate its own magnetic field
- As a result, the nucleus will experience a diminished magnetic field.
When the atom is bonded with an atom with a higher electronegativity, the electron density will be drawn away
- Thus DESHIELDING the nucleus, causing the nucleus to experience a stronger magnetic field.
When the atom is bonded with an atom with a lower electronegativity, the electron density will be drawn closer to the nucleus
- Thus SHIELDING the nucleus, causing the nucleus to experience a weaker magnetic field.
¶ Reporting Chemical Shift
Due to the effects of chemical shifting, the formula of calculating the resonance frequency needs to be adjusted
- is the sheilding constant
The higher the shielding effect, the larger the shielding constant
- The respective resonance frequency will decrease
The resonance frequency depends on the applied magnetic field, so it cannot be reported as an absolute value
- The value should be first converted to ppm ( parts per million )
- = chemical shift ( ppm )
- = Observed resonance frequency ( Hz )
- = Spectrometer operating frequency ( Hz )
- = Standard Resonance frequency ( Hz )
¶ Chemical Equivalence
An important consequnce of chemical shift is that every nuclei within the molecule will have a different value due to their relative position
- Each nuclei will give a unique peak in the spectrum
However, due to symmetry and the free rotation of sigma bond, some nuclei will exhibit chemical equivalence, and will not produce a new peak in the spectrum
- bonds restrict bond rotation and may result in multiple peaks for the same element as the equatorial and axial position have different chemical environment
- Ring flipping of cyclohexane may produce multiple peaks for the same element
- Molecules with trigonal bipyrimidal center may produce multiple peaks for the same element as the equatorial and axial position have different chemical environment ( The axial and equatorial position can interchange in high temperature, so multiple peaks can only be observed in low temperature )
Atoms of the same element can have different chemical shifts depending on their relationship with each other
- Homotopic and enantiotopic atoms have the same chemical shifts
Diastereotopic and heterotopic atoms have different chemical shiftsStereoisomerism matters in NMR spectroscopy
- Diastereomers have different electronic structureand therefore can be distinguished with NMR spectroscopy
- The corresponding nuclei in both enantiomeric environments have the exact same electronic surrounding, therefore enantiomers cannot be distinguished by NMR spectroscopy
Relative proportion of elements in molecules
The area under each NMR absorption can be electronically intergrated
- The relative number of elements in a molecule can be find by comparing the area under each peak
C-13 cannot be accurately intergrated due to its low abundencies
It is very unlikely to find more than one C-13 atom in the same molecule
- The relaxation time of C-13 differs depending on its bonding, causing their relative intensity to vary
¶ Spin-Spin Coupling
NMR active nuclei that are inequivalent will couple to each other
Their spin (including electrons ) will interact and influence the applied magnetic field experienced by each nuclei
- The nuclear spin states will influence the electron spin, which will in turn influence a neighboring spin active nucleus.
Coupling results in a splitting of peaks in the observed NMR spectrum
- The effect is transmitted through chemical bonds
- The effecacy of coupling decreases dramatically as the atoms separarting them increases ( coupling is usually significant when the coupling nuclei are only three bonds away from each other )
¶ Origin of Spin-Spin Coupling
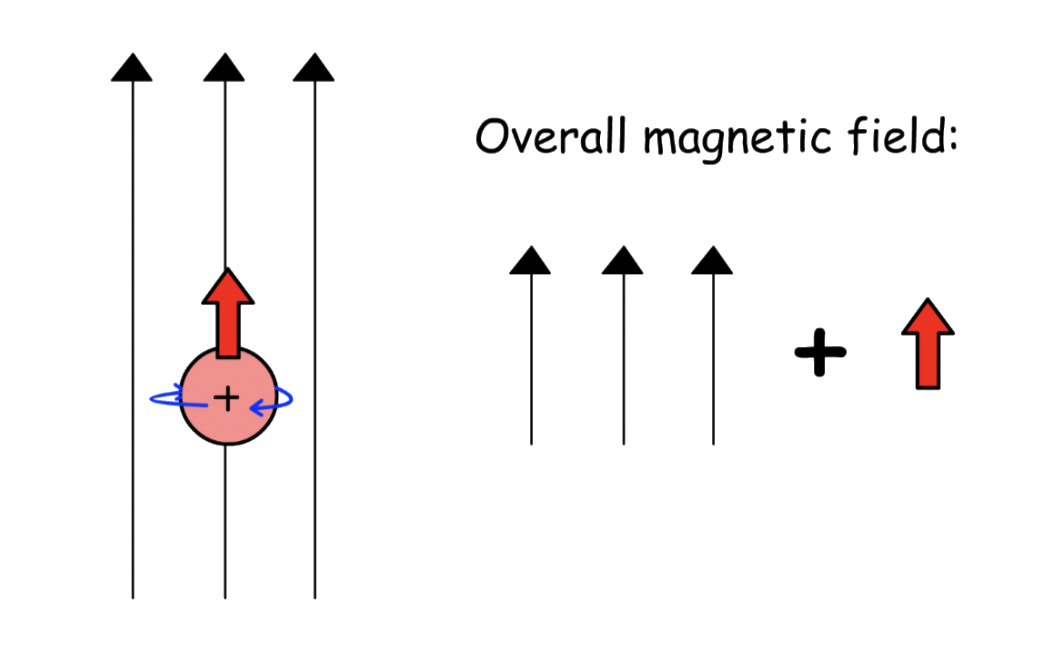
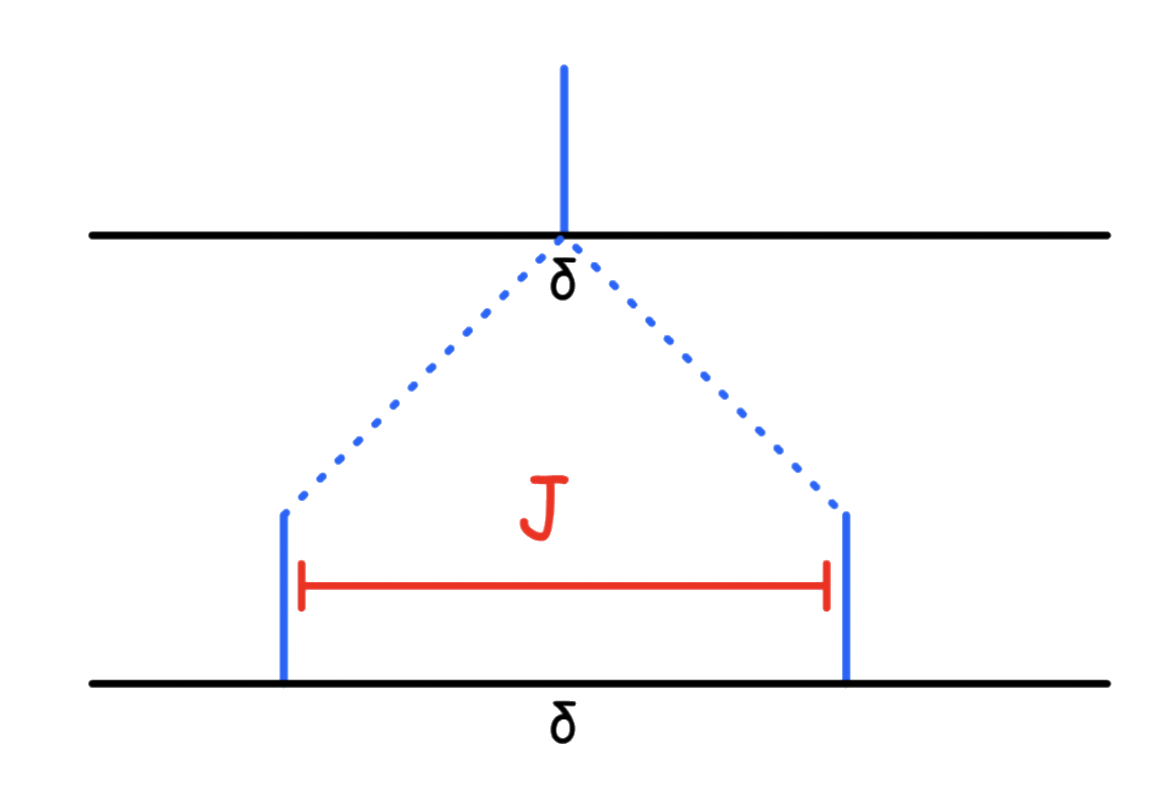
Under a magnetic field, a nucleus can either spin up or spin down
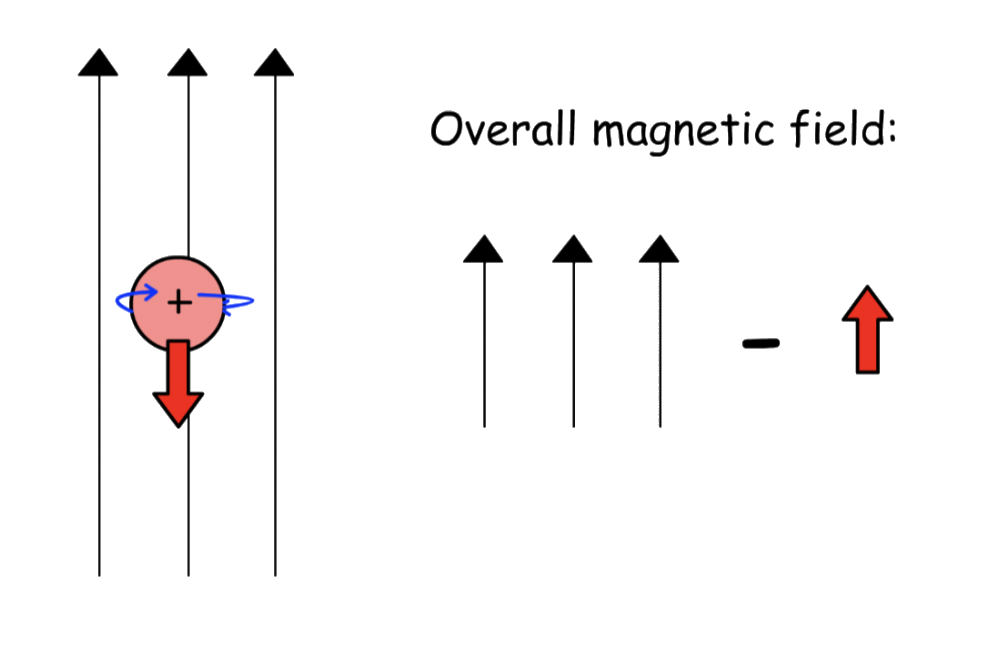
- When it is spinning up ( i.e. its own magnetic field is aligned with the applied magnetic field ), the overall magnetic field will be enhanced
- When it is spinning down ( i.e. its own magnetic field is against the applied magnetic field ), the overall magnetic field will be weakened
- If there is an NMR active nucleus nearby, it will experience a strengthened or weakened magnetic field
Since the transition energy is directly proportional to the strength of the magnetic field, the spin of the nucleus will affect the transition energy of the neighboring nuclei
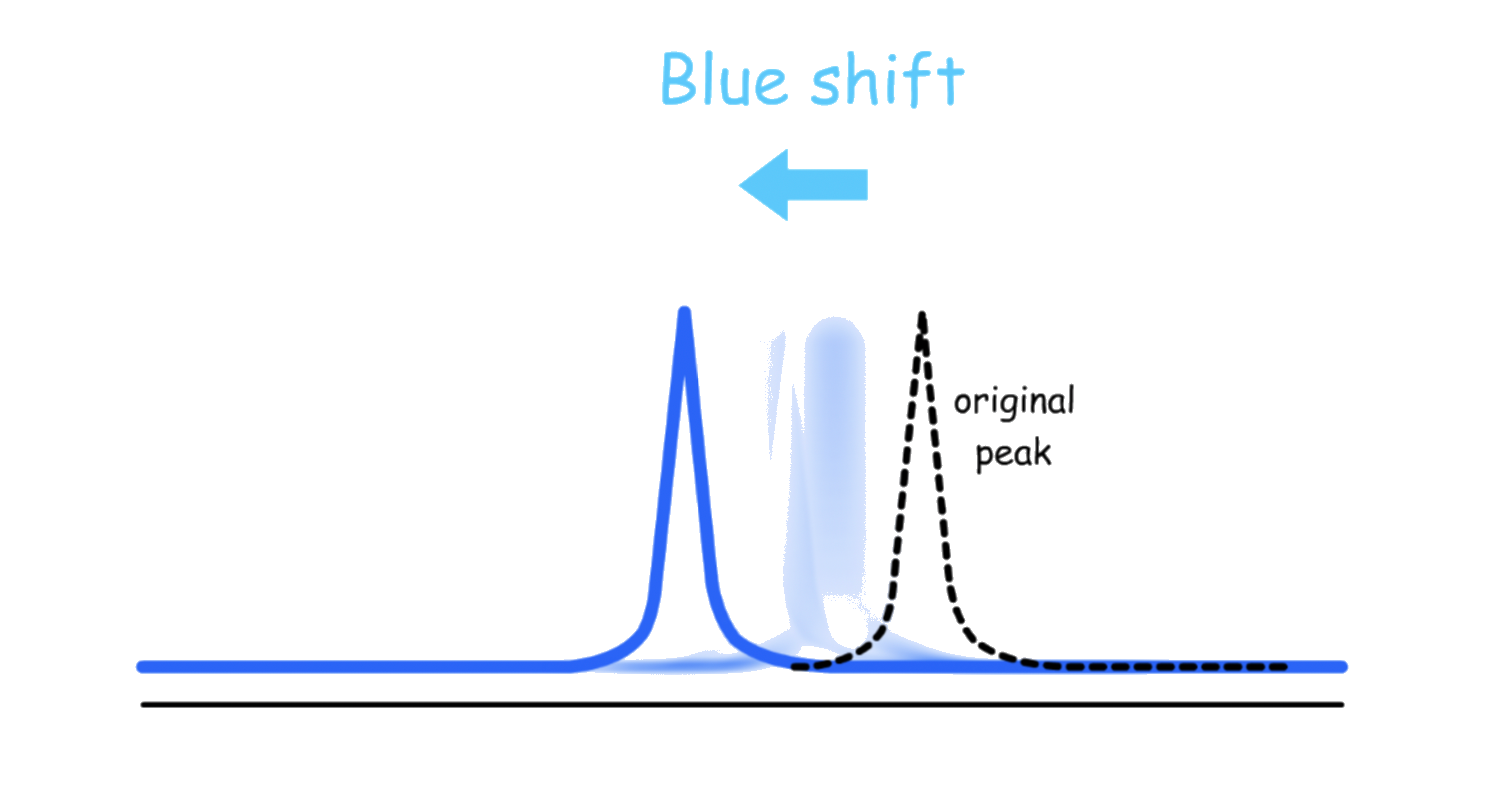
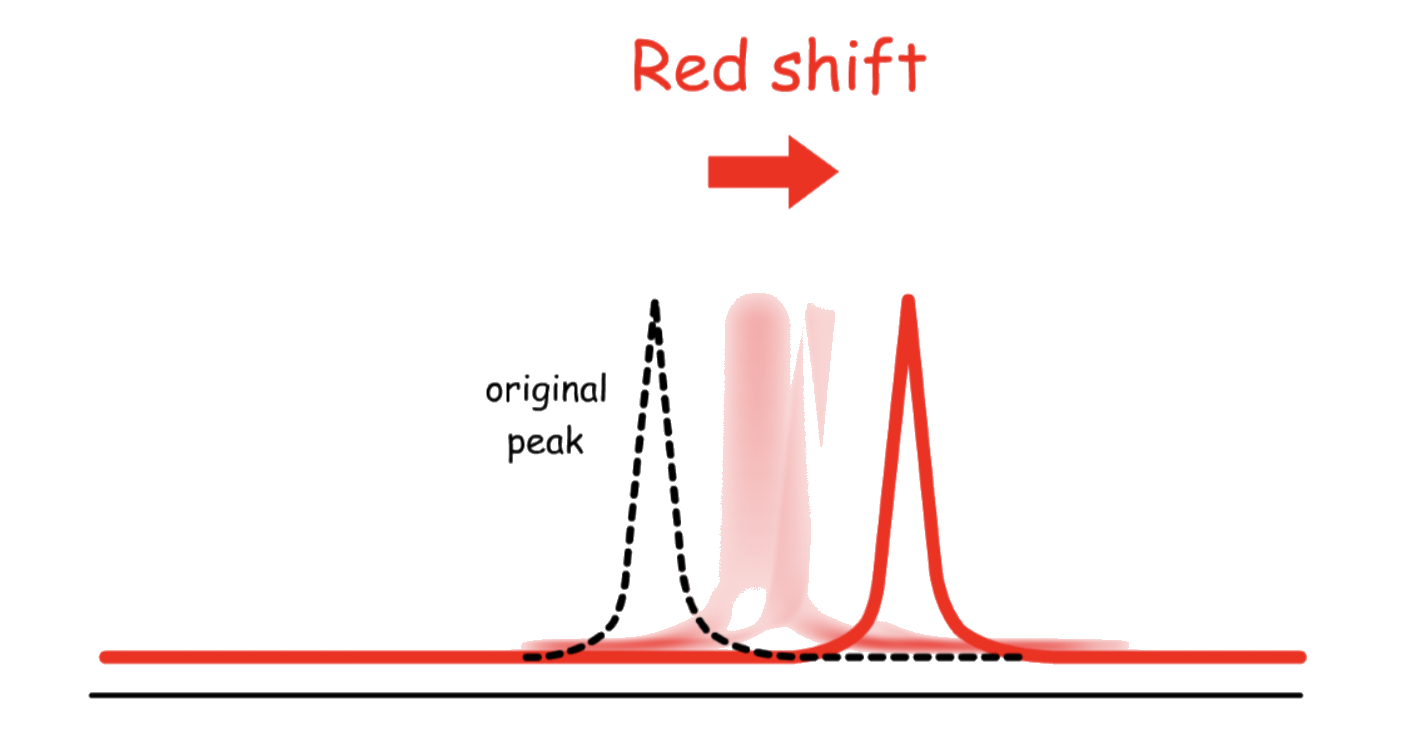
- When the magnetic field is enhanced, the transition energy will increase:
- When the magnetic field is weakened, the transition energy will decrease:
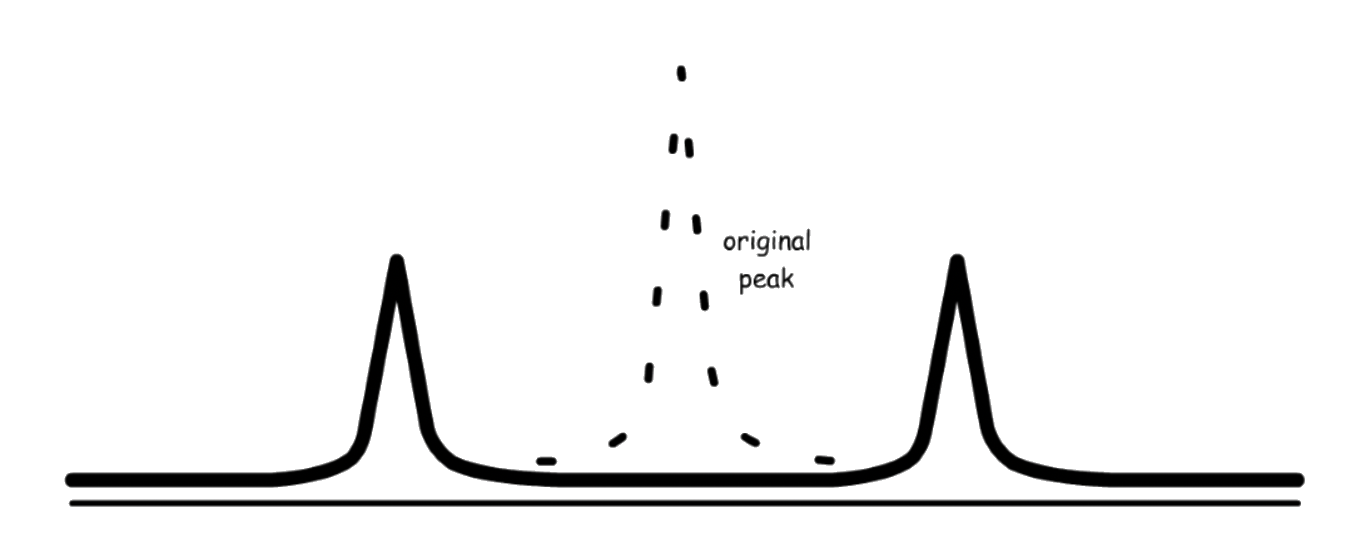
Since the nucleus can be either spinning up or down, both shifting will be occurring simultaneously
- We are effectively removing degeneracy of the system
- Note that the intensity of the peak is halved
-This effect is mutual between the coupled pair¶ Coupling Constant
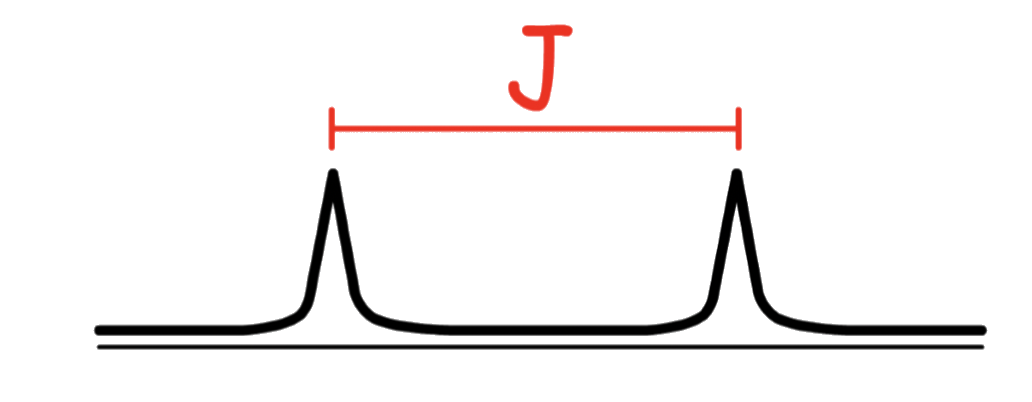
The coupling constant ( J ) is the distance between the peaks of a signal due to spin-spin coupling
The coupling constant is mutual to the coupled atoms
- If the nuclei is only coupling with a group of chemically equivalent atom(s), it will only have one coupling constant
- If the nuclei is coupling with chemically inequivalent atoms simultaneously, it will have multiple coupling constants, each corresponds to each coupling pair
The coupling constant is represented as such:
- n = number of bonds separating the nuclei
- A and X are the coupled atoms
Although the coupling constant is the distance between the peaks of the signal, we cannot measure it directly as its value varies between spectrometer
- The x-axis of the spectrum is in units of ppm
- To find the "universal" coupling constant, we must convert it to Hertz:
The magnitude of the coupling constant depends on several factors:
- Number of bonds separating nuclei
- As a rule of thumb 1J > 2J > 3J ( but not always the case )
- Product of their gyromagnetic ratio
- often heavier nuclei couple more strongly
- Larger when coupling with different elements
- Hybridization
- Larger when it has a larger s-character
- J is independent of the applied magnetic field
¶ Coupling with chemically equivalent nuclei
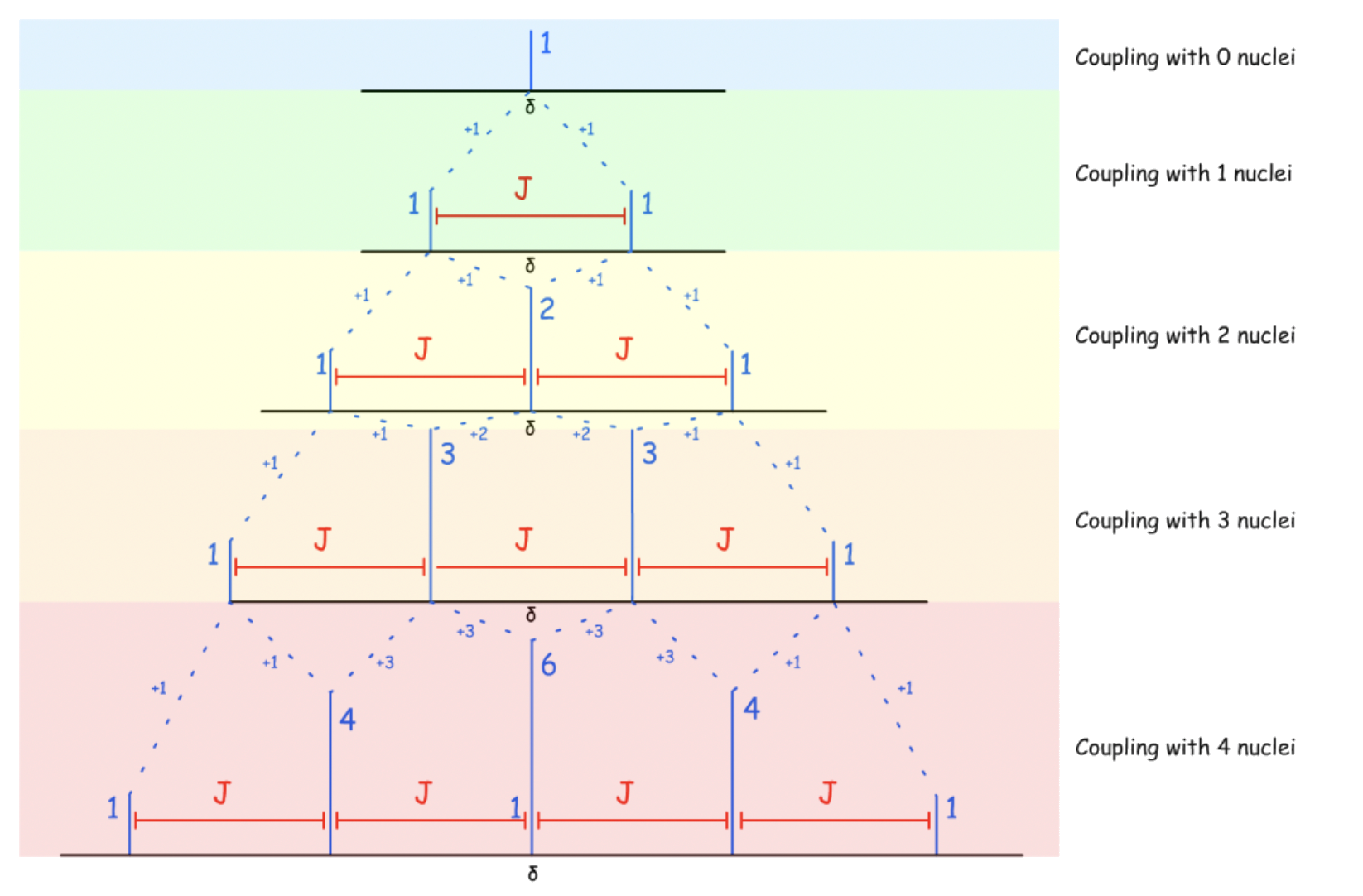
When a nuclei is coupling with another NMR active nuclei, its peak will split into 2I + 1 peaks
- For nuclei with , it will split into 2 peaks
When the nuclei is coupling with more than one chemically equivalent, the spitted peaks will split again
- When two peaks are in the same position, they will combine additively
Inequivalent spin combinations
We can also view it from the perspective of the number of inequivalent spin combinations.
- The number of different magnetic field strength experienced by the nucleus in question is equal to the number of inequivalent spin combinations the neighboring atom(s) can produce
- The intensity of the peak is the result of the ratio of identical spin combinations
This can be understood with a few examples
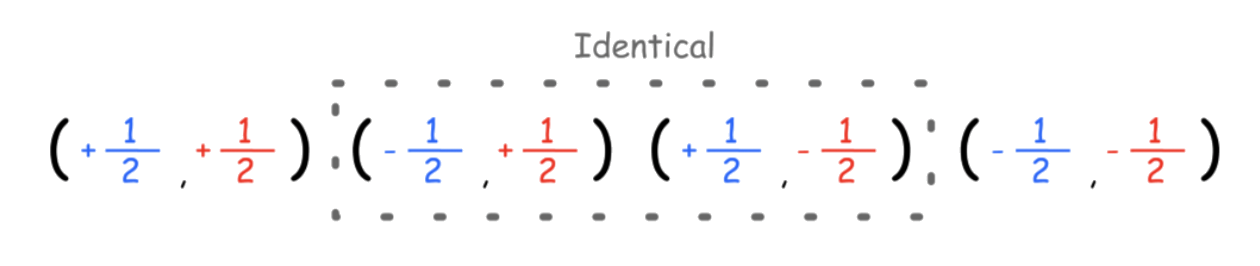
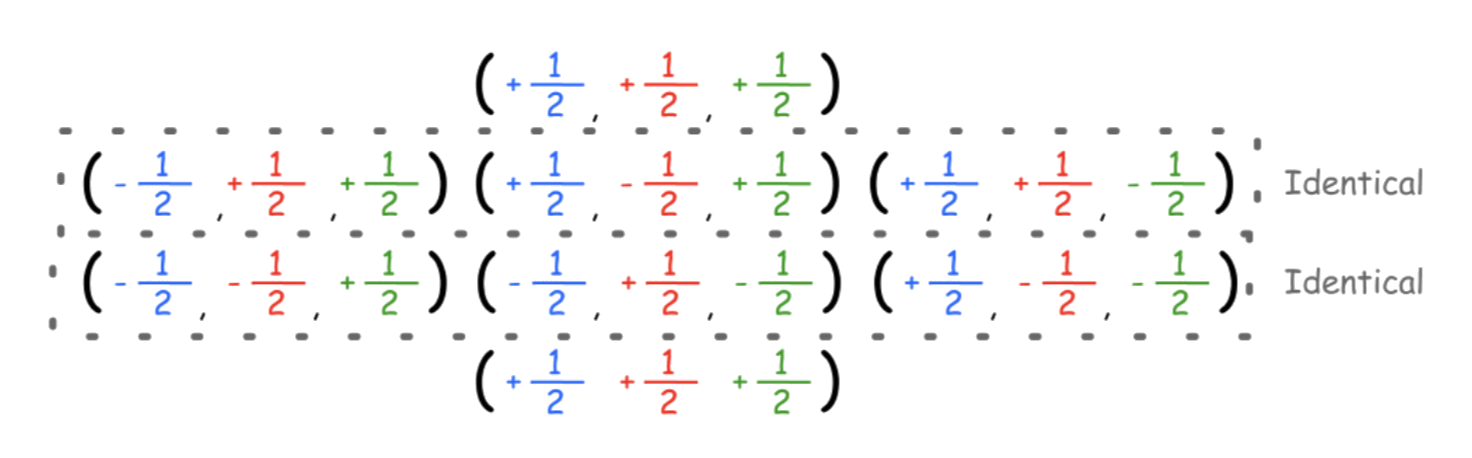
- When the nucleus is coupling with one nucleus, there are two distinct field combinations, which produces two peaks. Hence, the ratio of identical combinations are 1:1
- When the nucleus is coupling with two chemically equivalent nuclei, there are three distinct combinations, which produces three peaks. Hence, the ratio of identical combinations are 1:2:1
- When the nucleus is coupling with two chemically equivalent nuclei, there are four distinct combinations, which produces four peaks. Hence, the ratio of identical combinations are 1:3:3:1
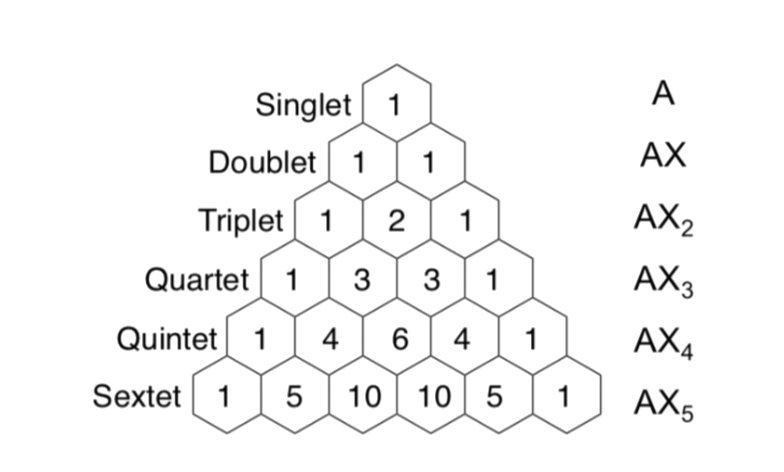
To simplify, the number of spitted peaks =
- n = Number of chemically equivalent coupled atoms
- I = The spin of the nuclei
For nuclei with , the number of splitted peaks and their relative intensities can be predicted from a Pascal's Triangle:
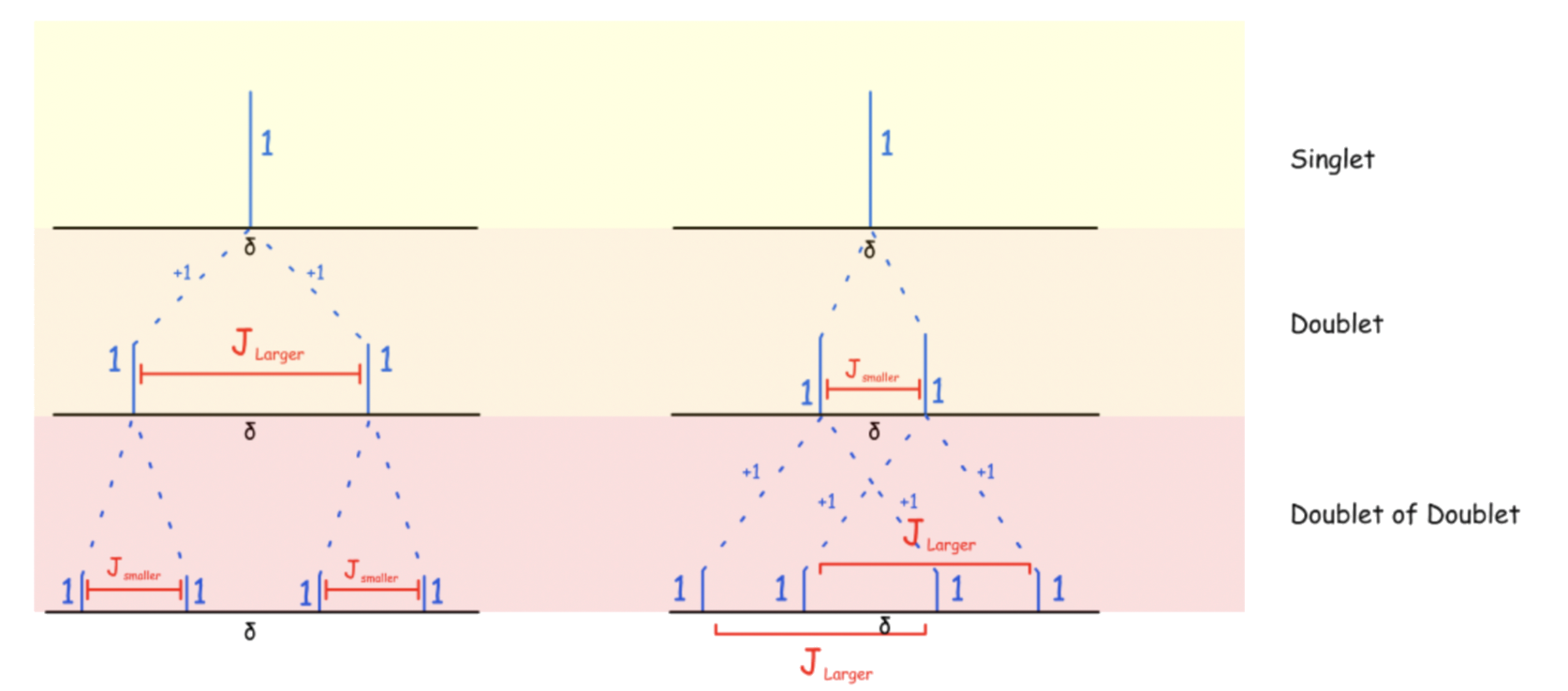
¶ Coupling with multiple chemically inequivalent species
When there is the nucleus is coupling with two chemically inequivalent species, the peaks will not combine, which results in a different phenomenon
For more complex situations, group the chemically equivalent species together first and find out each of their respective multiplicity, then combine them together
- i.e. XXXet of XXXet